二、希尔伯特空间
[希尔伯特(H)空间] 若无限维酉空间V中每个基本序列收敛于V中一个元素,则称V为完备的. 一个完备的无限维酉空间称为希尔伯特空间,或简称H空间.
在n维空间中的矢量定义为n个数![]() 的全体. 类似地,无限维空间中的矢量定义为t从a变到b的函数
的全体. 类似地,无限维空间中的矢量定义为t从a变到b的函数![]() .
.
矢量的加法与数乘定义为函数的加法与函数和数的乘法.
在H空间中两矢量的内积(数量积)公式为
(1)
[H空间的度量] 设![]() ,则
,则
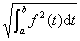
为矢量![]() 的长度. 设
的长度. 设![]() ,则矢量
,则矢量![]() 与
与![]() 之间的距离等于
之间的距离等于
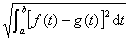
这个表达式称为函数![]() 与
与![]() 的均方差. 就是以均方差作为希尔伯特空间H中两元素间的距离的度量.
的均方差. 就是以均方差作为希尔伯特空间H中两元素间的距离的度量.
在H空间中两矢量![]() ,
,![]() 间的角度
间的角度![]() 定义为
定义为
(2)
因为对任意两个函数![]() 与
与![]() 都有不等式
都有不等式
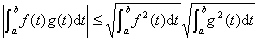
所以等式(2)的右端可以看作某角度![]() 的余弦.
的余弦.
[正交函数与正交函数系] 若非零矢量f与g的内积![]() ,则由(1)与(2)可知
,则由(1)与(2)可知![]() ,即
,即![]() .
因此称矢量f和g是正交的. 这时
.
因此称矢量f和g是正交的. 这时
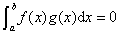
设![]() 表示两两正交的函数,而
表示两两正交的函数,而
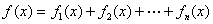
为它们的和,则![]() 的长度平方等于
的长度平方等于![]() 的长度平方和.
的长度平方和.
因为H空间中矢量的长度是用积分给出的,所以这时类似于商高定理由下面的公式给出:
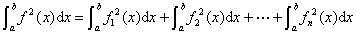
以上所述的积分,例如![]() ,是指勒贝格积分有意义而言的.
,是指勒贝格积分有意义而言的.
若H空间中函数系
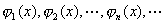
中的任意两函数相互正交,即
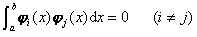
则称这个函数系为正交函数系. 若还满足
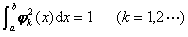
则称此函数系为标准正交系.
[依标准正交函数系的分解] 若在H空间中给定一个完备的标准正交函数系![]() (即不可能再加一个不恒为零的函数与系中的一切函数正交),则一切函数
(即不可能再加一个不恒为零的函数与系中的一切函数正交),则一切函数![]() 都可依这个系中函数展开成级数(平均收敛):
都可依这个系中函数展开成级数(平均收敛):
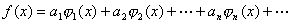
式中函数![]() 等于矢量
等于矢量![]() 在标准正交系中的矢量上的投影:
在标准正交系中的矢量上的投影:
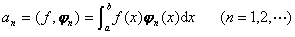
可以证明:
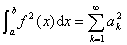
它的几何意义是,H空间中矢量的长度平方等于该矢量在完备的标准正交系中的矢量上的投影平方和.