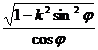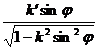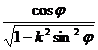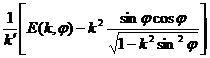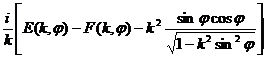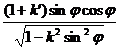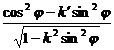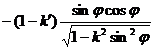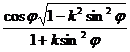Chapter 12 Special Functions
Special functions generally refer to functions for which the solution of a certain type of differential equation cannot be represented by a finite form of elementary functions . However, such functions are common in applications , such as Legendre functions , Bessel functions and many orthogonal polynomials, etc. ; Others are functions defined by specific forms of integrals , such as -functions , B - functions . There are also so-called elliptic functions , which are considered from the perspective of the periodicity of the function, which have nothing to do with differential equations . In addition to introducing these functions in this chapter In addition to the concept of functions, some expressions such as integrals, series and infinite products, asymptotic forms, relations between functions and their common properties are also given .
This chapter refers to the following symbols
where is a positive integer and is any number .
§ 1 Special functions defined by integrals
1.
Gamma function ( -function )
[ - definitions of functions and other expressions ]
The right-hand side is called the Euler integral of the second kind .
|
|
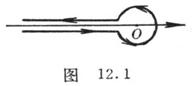
The integral route starts from infinity on the negative real axis , circles the origin in the positive direction, and then returns to the starting point (Figure 12.1 )
is a semi-pure function with a single pole, the corresponding residue is
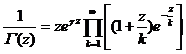
is called Euler's constant.
[ Function related formula ]
positive integer
very
(Remainder formula)
very
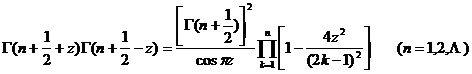
(multiplication formula)
(double formula)
[ - asymptotic expression of function ]
Stirling formula
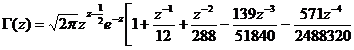
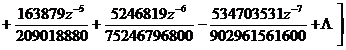
When it is a positive real number,
(i)
in the formula
(ii)
where is the Bernoulli number ( §7 ) .
[ Can be reduced to -integral of functions ]
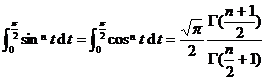
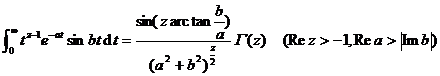
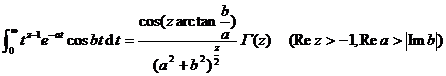
2.
Beta function ( B - function )
[ B - Definition of function and other expressions ]
The right-hand side is called the Euler integral of the first kind
[ B - the relevant formula of the function ]
positive integer
[ Integral into a B - function ]
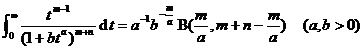
Third, the
Pusy function ( 
function )
[ Definition of functions and other expressions ]
( Gaussian integral formula )
( Dirichlet's formula )
where is Euler's constant .
[ Function related formula ]
[ special value of function ]
( for Euler's constant )
[ Asymptotic expression of function ]
where is the Bernoulli number ( § 7).
Fourth, the
Fresnel function
[ Definition of Fresnel function and other expressions ]
They are all integer functions .
in the formula 
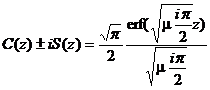
for the probability integral
[ Asymptotic expression of Fresnel function ]
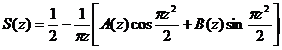
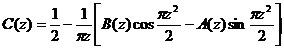
in the formula
very
5.
Probability integral ( error function )
[ Definition of Probability Integral and Series Expression ]
Probability integral ( or error function )
Co-probability integral ( or co-error function )
normal probability integral
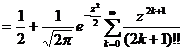
called the Kummer function )
[ Asymptotic Expression for Probability Integral ]
If the sum of the preceding terms of the series is used as an approximation , the error 
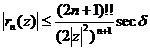
When it is a real number , its error does not exceed the absolute value of the first term omitted in the series .
At that time , .
Six,
sine integral and cosine integral
[ Definition of Sine Integral and Series Expression ]
They are all integer functions .
[ Definition and Series Expression of Cosine Integral ]
It is single-valued analysis in the plane with the semi- axes removed , where is Euler's constant .
definition
[ Relationship between functions ] When it is a real number , there are
[ asymptotic expression ]
in the formula 
especially
7.
Index Points
[ Definition of Exponential Integral and Other Expressions ]
It is single-valued analysis in the plane with the semi- axes removed , where is Euler's constant .
[ Asymptotic expression for exponential integral ]
in the formula
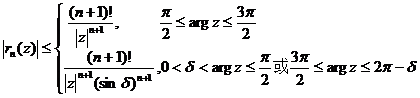
very
Eight,
logarithmic integral
[ Definition of Logarithmic Integral and Other Expressions ]
It resolves to a single value in the plane minus and .
where is Euler's constant .
[ Asymptotic Expression for Log Integral ]
in the formula
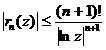
9.
Incomplete gamma function
[ Definition and other expressions of incomplete gamma function ]
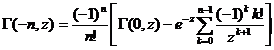
[ Related formula of incomplete gamma function ]
is not an integer , and
10.
Elliptic integrals
The [ elliptic integral ] has the form
Yes rational functions , yes cubic or quartic polynomials ) integrals are called elliptic integrals , which can be reduced to some integrals that can be represented by elementary functions .
[ Legendre Elliptic Integral ]
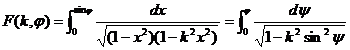
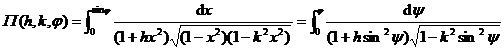
These three integrals are called Legendre elliptic integrals of the first, second, and third kinds, respectively . The numbers are called the modulus of these integrals , the numbers are called the complementary modulus , and the numbers are called the parameters of the third kind of integrals .
[ Weierstrass elliptic integral ]
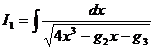
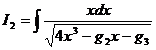
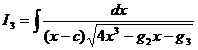
These three integrals are called Weylstrassian elliptic integrals of the first, second, and third kinds, respectively .
[ Complete Elliptic Integral ]
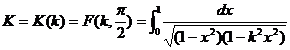
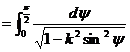
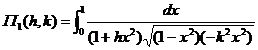
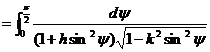
These three integrals are called complete elliptic integrals of the first, second, and third kinds respectively .
[ Series Expression of Elliptic Integral ]
+
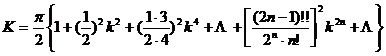
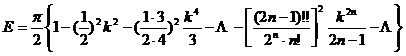
where is a hypergeometric series .
[ Formulas related to elliptic integral ]
is an integer )
is an integer )
( Legendre relation )
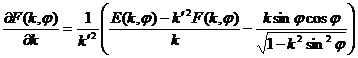
[ Elliptic integral replacement formula table ]
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
[ Complete elliptic integral replacement formula table ]
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
[ Integral that can be reduced to an elliptic integral ]
( set )
( set )
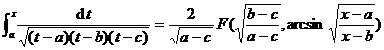( set )
( set )
( set )
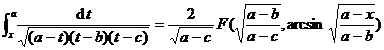(set )
( set )
(set )
(set )
(set )
(set )
(set )
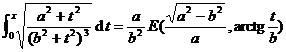(set )
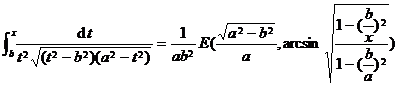(set )
