§ 6 Manifolds _    

    [ n -dimensional real manifold ] Assuming that M is a T ₂ connection space, M has a closed-capped open set family S , for each open set V S , there is a topological transformation f _V to change V to an n -dimensional interval, then it is called { f _V | V S } is an n -dimensional real manifold structure of M, and M is said to be an n -dimensional real manifold .  

    [ Local coordinate method ] Assuming that { f _V | V S } is a manifold structure of manifold M , then each V S is called a coordinate region . Each f _V is a local coordinate method in V, and for each point x V ,  

Called the coordinate of x , the real number x _k ( k =1, × × × , n ) is called the kth coordinate of x .

    [ Connection relationship ]  Assuming V S , V' S , V ∩ V' 1 φ , then each point x V ∩ V' has a coordinate sum under the two local coordinate methods of f _V and f _V ' , and their relationship can be expressed as Expressed as

                     (1)

From the definition of manifold, it is the topological transformation of changing f _V ( V ∩ V' ) to f _V' ( V ∩ V' ), which is called the connection relationship from the local coordinate method f _V to the local coordinate method f _V' .

    [ Differential Structure and Differential Manifold ]  Assume { f _V | V S } is a manifold structure of manifold M , which is the connection relationship from f _V to f _V' . Expression (1) can be rewritten as a system of equations

                             ( 2)

If each function in (2) exists in f _V ( V ∩ V' ) with respect to real variables x ₁ , × × × , x _n in the order of 1 to m partial derivatives exist and are continuous, then it is called m -order continuously differentiable . If each partial derivative of each order exists (and therefore is continuous) in f V ( V ∩ V' ), then it is said to _be continuously differentiable of order ∞ . If each is in f _V ( V ∩ V' ) parsed in (i.e. at every point < x ₁ ⁰ , × × ×, x _n ⁰ > ( f _V ( V ∩ V' )) in a neighborhood , ( x ₁ , × × × , x _n ) can be expanded into a power series of n real variables ), then it is called analytical or ω -order continuously differentiable .

    A manifold structure { f _V | V S } is called an m -order differential structure if all the articulation relations of it are m -order continuously differentiable (and therefore are invertible m -order continuously differentiable) . If a manifold All connections of a structure are continuously differentiable of order ∞, then it is called a differential structure of order ∞ . If all connections of a manifold structure are analytic, then it is called an analytic structure .

    Assuming that a manifold structure { f _V | V S } of a real manifold M is an m -order differential structure or an infinity-order differential structure or a real analytical structure, then M is an m -order differential manifold or an infinity-order under this structure, respectively. Differential manifolds or real analytical manifolds .

    [ The equivalence of differential structure ] Suppose { f _V | V S } is an m -order differential structure of the manifold M. Also suppose that G is an open set in M , and f is a function defined in G. For each point x G ∩ V ( V S ), f ( x ) can be expressed as  

f ( x ) = ( )

Assuming that the partial derivatives of each order from 1 to k ( 0 km ) are continuous with respect to these n real variables , then f is said to be k -order continuous differentiable in G ∩ V. If f is in every G ∩ V ( V S ) can be continuously differentiated at level k , then f can be continuously differentiated at level k in G , denoted as f C _k ( G ), since all the connection relationships of { f _V | V S } can be continuously differentiated at level m , and assuming that 0 k m , such a definition above does not contradict any x V ∩ V' ( V S , V' S ) .

    Let { f _V | V S } and { f _W | W π } be two m -order differential structures of a manifold M (where π is also a closed-open family of M) . If { f V _| V S } ∪ { f _W | W π } is an m -order differential structure of M , then { f _V | V S } and { f _W | W π } are said to be equivalent . The manifold M The necessary and sufficient conditions for the equivalence of the two m -order differential structures of are: for any open set G in M , the function families C _k ( G ) ( k = 0, 1, × × × , m ) determined by them are consistent .

    The concept of the equivalence of two ∞ order differential structures or the equivalence of two real analytic structures of a manifold M can also be similarly defined .

    [ Orientable Manifold ]  Suppose that a neighborhood of a point in n -dimensional real space is transformed into a neighborhood of a point by a topological transformation f , that is, f ( ) = . If f is reversibly differentiated continuously, and the Jacobian formula

                    

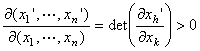
Then say f to hold the stance at this point .

    If f is not differentiable, substituting the difference quotient (see Chapter 5) for the partial derivative also allows f to hold the pose at one point .

    Assuming that the manifold M has a manifold structure, any of its connection relations maintains a posture at each point in the respective defining open set, then the manifold structure is called a directional manifold structure, and the manifold M is defined by it. towards .

    Assuming that { f _V | V S } and { f _W | W π } are two directed structures of the manifold M , and { f _V | V S }∪{ f _W | W π } is also a directed structure of M , then They are said to be oriented in the same direction .

    Suppose { f _V | V S } is a directed structure of the manifold M , and suppose

                       f _V ( x ) = x    V

then

g _V ( x ) = x   V

is another directional structure { g _V | V S } . Obviously { f _V | V S }∪{ g _V | V S } is no longer a directional structure . Then we call { f _V | V S } and { g _V | V S } is in the opposite direction .

    Therefore, if the manifold M has a directional structure, then M has two types of directional structures, the structures of the same type are oriented in the same direction, and the directional structures of different types are opposite . Therefore, when the type of directional structure is not specified, it is only said that there are The manifold M of a directional structure is orientable .

    It can be proved that any manifold structure of an orientable manifold can become a directional structure as long as a part of the local coordinate method is modified as in the above specification g _V , and the orientation can be consistent with this type of directional structure, or it can be consistent with another directional structure. class consistency .

    Therefore, although the differential structures of an orientable differential manifold are not all oriented, each equivalence class of differential structures must contain two directional structures, and the orientations are opposite to each other .

The simplest example of an unorientable manifold is the " Möbius belt", which is a one-sided surface whose model can be obtained by     twisting a rectangular piece of paper 180 ° , and aligning the sides ad and cb is glued together, a and c are superimposed, b and d are superimposed .

 [ Complex Analytical Manifold ] Rewrite the point < x ₁ , x ₂ > in  the two-dimensional real space R ^{2 as a complex number} x ₁ + ix ₂ , then we get the one-dimensional complex space C ¹ , and C ¹ is the general topology of R ² When topology . The general topology of R ² can use the whole of two-dimensional intervals as the basis, or the whole of open circles as the basis . In C ¹ , for the convenience of notation, it is more common to use the latter as the basis . A complex number z ₀ as the center An open circle can be expressed as { z | z C ¹ and | z -z ₀ |< r }, where the radius r is a positive number . The topological product of n C 1s is called an n - dimensional ^complex space C n ^, and a basis of the topology of C ⁿ is the direct product of n open circles in C ¹ The totality of , the direct product of n open circles is called an n -fold column .

    In the definition of manifold, if " n -dimension interval" is changed to " n -column", it becomes the definition of "complex manifold" .

    The complex analytic structure of a complex manifold is defined in the same way as the real analytic structure of a real manifold . In particular, one-dimensional complex analytic manifolds are called Riemann surfaces, which are an important concept in the theory of complex functions (see Chapter 10) .

    [ The Theorem of Existence ]

    Theorem 1  Some manifolds cannot have a first-order differential structure .

    Note that when m m' 1, by definition, the m -order differential structure must be the m' -order differential structure, so the manifold mentioned in Theorem 1 must not have any order of differential structure .

    Theorem 2 An m ( m 1 )-level differential structure of the second countable real manifold must have an equivalent ∞-level differential structure (equivalent here means to treat it as an m -level differential structure) .  

    Theorem 3 The sphere in 8    -dimensional Euclidean space has an unequal differential structure .

It is known from Theorem 3 that manifolds of unequal differential structures do exist . As for the knowledge of the differential structure problem of the sphere itself, it has now been proved that the number of different equivalence classes of each differential structure dn _is equal to some The number of elements of a finite group, and there are many d _n have been calculated, such as

n	1	2	3	4	5	6	7	8	9	10	11	12	13	14	15
d n	1	1	?	1	1	1	28	2	8	6	992	1	3	2	16256

It can be seen from the table that there are a total of 28 types of differential structures, and the different types are not equivalent . d ₃ , that is , the number of differential structures that are not equivalent, has not been calculated .

    Theorem 4 Any m ( 1 m ∞ ) order differential structure of the second countable and orientable two-dimensional real manifold is equivalent to a complex analytic structure (the latter is regarded as an m order real differential structure) .