|
|
|
N.B. A detailed on-line essay by S. Finch was the starting point for this entry.
Also called a Sidon Sequence. An Infinite Sequence of Positive Integers
| (1) |
| (2) |
Zhang (1993, 1994) showed that
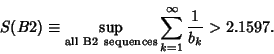 |
(3) |
See also A-Sequence, Mian-Chowla Sequence
References
Finch, S. ``Favorite Mathematical Constants.'' http://www.mathsoft.com/asolve/constant/erdos/erdos.html
Guy, R. K. ``Packing Sums of Pairs,'' ``Three-Subsets with Distinct Sums,'' and ``
Sloane, N. J. A. Sequence
A005282/M1094
in ``An On-Line Version of the Encyclopedia of Integer Sequences.''
http://www.research.att.com/~njas/sequences/eisonline.html and Sloane, N. J. A. and Plouffe, S.
The Encyclopedia of Integer Sequences. San Diego: Academic Press, 1995.
Zhang, Z. X. ``A B2-Sequence with Larger Reciprocal Sum.'' Math. Comput. 60, 835-839, 1993.
Zhang, Z. X. ``Finding Finite B2-Sequences with Larger
![]() -Sequences,'' and
-Sequences,'' and
![]() -Sequences Formed by the Greedy Algorithm.'' §C9, C11, E28, and E32
in Unsolved Problems in Number Theory, 2nd ed.
New York: Springer-Verlag, pp. 115-118, 121-123, 228-229, and 232-233, 1994.
-Sequences Formed by the Greedy Algorithm.'' §C9, C11, E28, and E32
in Unsolved Problems in Number Theory, 2nd ed.
New York: Springer-Verlag, pp. 115-118, 121-123, 228-229, and 232-233, 1994.
![]() .'' Math. Comput. 63, 403-414, 1994.
.'' Math. Comput. 63, 403-414, 1994.