A distribution with probability function
where 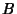 is a Beta Function. The Mode of a variate distributed as
is a Beta Function. The Mode of a variate distributed as
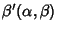 is
is
If 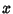 is a
is a
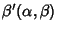 variate, then
variate, then  is a
is a
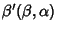 variate. If
variate. If 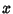 is a
is a
 variate, then
variate, then 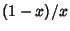 and
and 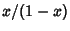 are
are
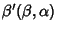 and
and
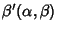 variates. If
variates. If 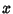 and
and  are
are
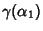 and
and
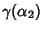 variates, then
variates, then 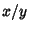 is a
is a
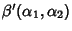 variate. If
variate. If  and
and  are
are 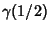 variates, then
variates, then
 is a
is a
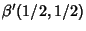 variate.
variate.
© 1996-9 Eric W. Weisstein
1999-05-26