|
|
|
N.B. A detailed on-line essay by S. Finch was the starting point for this entry.
Let ![]() be the set of Complex analytic functions
be the set of Complex analytic functions ![]() defined on an open region containing the closure of the unit disk
defined on an open region containing the closure of the unit disk
![]() satisfying
satisfying ![]() and
and ![]() . For each
. For each ![]() in
in ![]() , let
, let ![]() be the Supremum of all
numbers
be the Supremum of all
numbers ![]() such that there is a disk
such that there is a disk ![]() in
in ![]() on which
on which ![]() is One-to-One and such that
is One-to-One and such that ![]() contains a disk
of radius
contains a disk
of radius ![]() . In 1925, Bloch (Conway 1978) showed that
. In 1925, Bloch (Conway 1978) showed that ![]() . Define Bloch's constant by
. Define Bloch's constant by
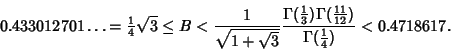
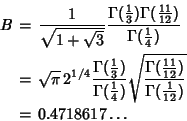
See also Landau Constant
References
Conway, J. B. Functions of One Complex Variable, 2nd ed. New York: Springer-Verlag, 1989.
Finch, S. ``Favorite Mathematical Constants.'' http://www.mathsoft.com/asolve/constant/bloch/bloch.html
Le Lionnais, F. Les nombres remarquables. Paris: Hermann, p. 25, 1983.
Minda, C. D. ``Bloch Constants.'' J. d'Analyse Math. 41, 54-84, 1982.