Bourget Function

$$J_{n,k}(z) = {1\over \pi i}\int t^{-n-1}\left({t+{1\over t}}\right)^k\mathop{\... ...\nolimits \left[{{\textstyle{1\over 2}}z\left({t-{1\over t}}\right)}\right]\,dt$$

$$= {1\over \pi}\int_0^\pi (2\cos\theta)^k\cos(n\theta-z\sin\theta)\,d\theta.$$

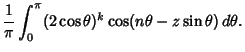See also Bessel Function of the First Kind

References

Hazewinkel, M. (Managing Ed.). Encyclopaedia of Mathematics: An Updated and Annotated Translation of the Soviet ``Mathematical Encyclopaedia.'' Dordrecht, Netherlands: Reidel, p. 465, 1988.