Cannonball Problem

Find a way to stack a Square of cannonballs laid out on the ground into a Square Pyramid (i.e., find a Square Number which is also Square Pyramidal). This corresponds to solving the Diophantine Equation

$$\sum_{i=1}^k i^2 = {\textstyle{1\over 6}}k(1+k)(1+2k) = N^2$$

for some pyramid height $k$. The only solution is $k=24$, $N=70$, corresponding to 4900 cannonballs (Ball and Coxeter 1987, Dickson 1952), as conjectured by Lucas (1875, 1876) and proved by Watson (1918).

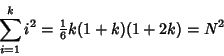
See also Sphere Packing, Square Number, Square Pyramid, Square Pyramidal Number

References

Ball, W. W. R. and Coxeter, H. S. M. Mathematical Recreations and Essays, 13th ed. New York: Dover, p. 59, 1987.

Dickson, L. E. History of the Theory of Numbers, Vol. 2: Diophantine Analysis. New York: Chelsea, p. 25, 1952.

Lucas, É. Question 1180. Nouvelles Ann. Math. Ser. 2 14, 336, 1875.

Lucas, É. Solution de Question 1180. Nouvelles Ann. Math. Ser. 2 15, 429-432, 1876.

Ogilvy, C. S. and Anderson, J. T. Excursions in Number Theory. New York: Dover, pp. 77 and 152, 1988.

Pappas, T. ``Cannon Balls & Pyramids.'' The Joy of Mathematics. San Carlos, CA: Wide World Publ./Tetra, p. 93, 1989.

Watson, G. N. ``The Problem of the Square Pyramid.'' Messenger. Math. 48, 1-22, 1918.