|
|
|
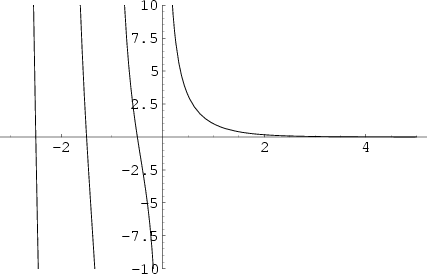

The central beta function is defined by
| (1) |
| (2) | |||
| (3) | |||
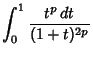 |
(4) | ||
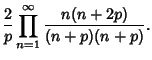 |
(5) |
| (6) |
| (7) |
| (8) |
| (9) |
| (10) |
| (11) |
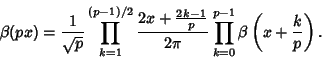 |
(12) |
See also Beta Function, Regularized Beta Function
References
Borwein, J. M. and Zucker, I. J. ``Elliptic Integral Evaluation of the Gamma Function at Rational Values of Small
Denominators.'' IMA J. Numerical Analysis 12, 519-526, 1992.
|
|
|
© 1996-9 Eric W. Weisstein