|
|
|
Using a Chebyshev Polynomial of the First Kind ![]() , define
, define
![\begin{eqnarray*}
c_j&\equiv& {2\over N}\sum_{k=1}^N f(x_k)T_j(x_k)\\
&=& {2\...
...ght]\cos\left\{{\pi j(k-{\textstyle{1\over 2}})\over N}\right\}.
\end{eqnarray*}](c1_1266.gif)
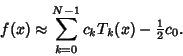
References
Press, W. H.; Flannery, B. P.; Teukolsky, S. A.; and Vetterling, W. T. ``Chebyshev Approximation,''
``Derivatives or Integrals of a Chebyshev-Approximated Function,'' and ``Polynomial Approximation from
Chebyshev Coefficients.'' §5.8, 5.9, and 5.10 in
Numerical Recipes in FORTRAN: The Art of Scientific Computing, 2nd ed. Cambridge, England:
Cambridge University Press, pp. 184-188, 189-190, and 191-192, 1992.