or
where 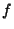 is a Function of one variable and
is a Function of one variable and 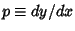 . The general solution is
. The general solution is 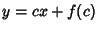 . The singular
solution Envelopes are
. The singular
solution Envelopes are 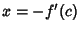 and
and 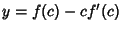 .
.
See also d'Alembert's Equation
References
Boyer, C. B. A History of Mathematics. New York: Wiley, p. 494, 1968.
© 1996-9 Eric W. Weisstein
1999-05-26