Colorable

Color each segment of a Knot Diagram using one of three colors. If

1. at any crossing, either the colors are all different or all the same, and

2. at least two colors are used,

then a Knot is said to be colorable (or more specifically, Three-Colorable). Colorability is invariant under Reidemeister Moves, and can be generalized. For instance, for five colors 0, 1, 2, 3, and 4, a Knot is five-colorable if

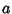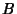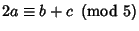1. at any crossing, three segments meet. If the overpass is numbered $a$ and the two underpasses $B$ and $C$, then $2a\equiv b+c\ \left({{\rm mod\ } {5}}\right)$, and

2. at least two colors are used.

Colorability cannot alway distinguish Handedness. For instance, three-colorability can distinguish the mirror images of the Trefoil Knot but not the Figure-of-Eight Knot. Five-colorability, on the other hand, distinguishes the Mirror Images of the Figure-of-Eight Knot but not the Trefoil Knot.

See also Coloring