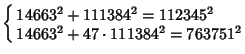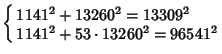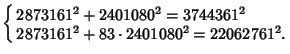A concordant form is an integer Triple 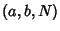 where
where
with 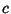 and
and 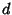 integers. Examples include
Dickson (1962) states that C. H. Brooks and S. Watson found in The Ladies' and Gentlemen's Diary (1857) that
integers. Examples include
Dickson (1962) states that C. H. Brooks and S. Watson found in The Ladies' and Gentlemen's Diary (1857) that
 and
and 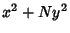 can be simultaneously squares for
can be simultaneously squares for 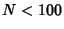 only for 1, 7, 10, 11, 17, 20, 22, 23, 24, 27,
30, 31, 34, 41, 42, 45, 49, 50, 52, 57, 58, 59, 60, 61, 68, 71, 72, 74, 76, 77, 79, 82, 85, 86, 90, 92, 93, 94, 97, 99,
and 100 (which evidently omits 47, 53, and 83 from above). The list of concordant primes less than 1000 is now complete
with the possible exception of the 16 primes 103, 131, 191, 223, 271, 311, 431, 439, 443, 593, 607, 641, 743, 821,
929, and 971 (Brown).
only for 1, 7, 10, 11, 17, 20, 22, 23, 24, 27,
30, 31, 34, 41, 42, 45, 49, 50, 52, 57, 58, 59, 60, 61, 68, 71, 72, 74, 76, 77, 79, 82, 85, 86, 90, 92, 93, 94, 97, 99,
and 100 (which evidently omits 47, 53, and 83 from above). The list of concordant primes less than 1000 is now complete
with the possible exception of the 16 primes 103, 131, 191, 223, 271, 311, 431, 439, 443, 593, 607, 641, 743, 821,
929, and 971 (Brown).
See also Congruum
References
Brown, K. S. ``Concordant Forms.''
http://www.seanet.com/~ksbrown/kmath286.htm.
Dickson, L. E. History of the Theory of Numbers, Vol. 1: Divisibility and Primality.
New York: Chelsea, p. 475, 1952.
© 1996-9 Eric W. Weisstein
1999-05-26
![]() where
where