Cumulant-Generating Function

Let $M(h)$ be the Moment-Generating Function. Then

$$K(h)\equiv\ln M(h) =\kappa_1 h+{\textstyle{1\over 2!}} h^2\kappa_2+{\textstyle{1\over 3!}} h^3\kappa_3+\ldots.$$

If

$$L=\sum_{j=1}^M c_jx_j$$

is a function of $N$ independent variables, the cumulant generating function for $L$ is then

$$K(h)=\sum_{j=1}^N K_j(c_j h).$$

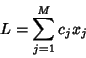
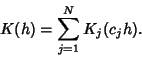
See also Cumulant, Moment-Generating Function

References

Abramowitz, M. and Stegun, C. A. (Eds.). Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, p. 928, 1972.

Kenney, J. F. and Keeping, E. S. ``Cumulants and the Cumulant-Generating Function'' and ``Additive Property of Cumulants.'' §4.10-4.11 in Mathematics of Statistics, Pt. 2, 2nd ed. Princeton, NJ: Van Nostrand, pp. 77-80, 1951.