The probability of getting at least one ``6'' in four rolls of a single 6-sided Die is
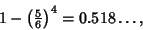 |
(1) |
which is slightly higher than the probability of at least one double 6 in 24 throws,
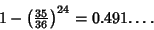 |
(2) |
de Mere suspected that (1) was higher than (2). He posed the question to Pascal,  who solved the problem and
proved de Mere correct.
who solved the problem and
proved de Mere correct.
See also Dice
References
Kraitchik, M. ``A Dice Problem.'' §6.2 in Mathematical Recreations. New York: W. W. Norton, pp. 118-119, 1942.
© 1996-9 Eric W. Weisstein
1999-05-24