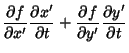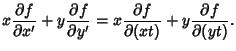Let 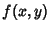 be a Homogeneous Function of order
be a Homogeneous Function of order 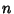 so that
so that
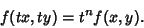 |
(1) |
Then define 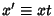 and
and 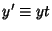 . Then
. Then
Let 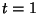 , then
, then
 |
(3) |
This can be generalized to an arbitrary number of variables
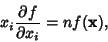 |
(4) |
where Einstein Summation has been used.
© 1996-9 Eric W. Weisstein
1999-05-25
![]() be a Homogeneous Function of order
be a Homogeneous Function of order ![]() so that
so that