|
|
|
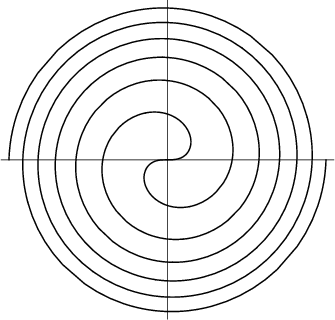
An Archimedean Spiral with ![]() having polar equation
having polar equation
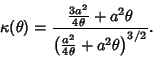
References
Dixon, R. Mathographics. New York: Dover, p. 121, 1991.
Gray, A. Modern Differential Geometry of Curves and Surfaces. Boca Raton, FL: CRC Press, pp. 69-70, 1993.
Lee, X. ``Equiangular Spiral.''
http://www.best.com/~xah/SpecialPlaneCurves_dir/EquiangularSpiral_dir/equiangularSpiral.html.
Lockwood, E. H. A Book of Curves. Cambridge, England: Cambridge University Press, p. 175, 1967.
MacTutor History of Mathematics Archive. ``Fermat's Spiral.''
http://www-groups.dcs.st-and.ac.uk/~history/Curves/Fermats.html.
Wells, D. The Penguin Dictionary of Curious and Interesting Geometry. Middlesex, England: Penguin Books, 1991.