|
|
|
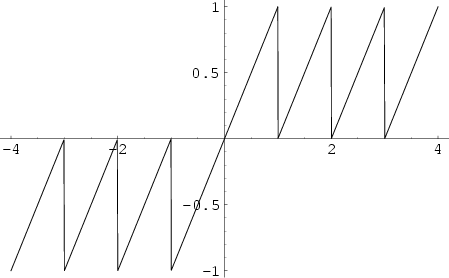
The function giving the fractional (nonintegral) part of a number and defined as
See also Ceiling Function, Floor Function, Nint, Round, Truncate, Whole Number
References
Spanier, J. and Oldham, K. B. ``The Integer-Value Int(
![]() ) and Fractional-Value frac(
) and Fractional-Value frac(![]() ) Functions.''
Ch. 9 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 71-78, 1987.
) Functions.''
Ch. 9 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 71-78, 1987.