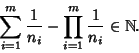Giuga Sequence

A finite, increasing sequence of Integers 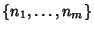$\{n_1, \ldots, n_m\}$ such that

$$\sum_{i=1}^m {1\over n_i}-\prod_{i=1}^m{1\over n_i}\in\Bbb{N}.$$

A sequence is a Giuga sequence Iff it satisfies

$$n_i\vert(n_1\cdots n_{i-1}\cdot n_{i+1}\cdot n_m-1)$$

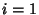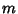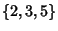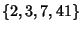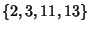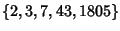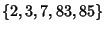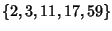for $i=1$, ..., $m$. There are no Giuga sequences of length 2, one of length 3 ($\{2, 3, 5\}$), two of length 4 ( $\{2, 3, 7, 41\}$ and $\{2, 3, 11, 13\}$), 3 of length 5 ( $\{2, 3, 7, 43, 1805\}$, $\{2, 3, 7, 83, 85\}$, and $\{2, 3, 11, 17, 59\}$), 17 of length 6, 27 of length 7, and hundreds of length 8. There are infinitely many Giuga sequences. It is possible to generate longer Giuga sequences from shorter ones satisfying certain properties.

See also Carmichael Sequence

References

Borwein, D.; Borwein, J. M.; Borwein, P. B.; and Girgensohn, R. ``Giuga's Conjecture on Primality.'' Amer. Math. Monthly 103, 40-50, 1996.