|
|
|
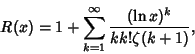
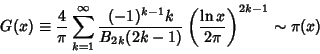
References
Berndt, B. C. Ramanujan's Notebooks, Part IV. New York: Springer-Verlag, pp. 124-129, 1994.
Gram, J. P. ``Undersøgelser angaaende Maengden af Primtal under en given Graeense.''
K. Videnskab. Selsk. Skr. 2, 183-308, 1884.
Ingham, A. E. Ch. 5 in The Distribution of Prime Numbers. New York: Cambridge, 1990.
Vardi, I. Computational Recreations in Mathematica. Reading, MA: Addison-Wesley, p. 74, 1991.