The equations defined by
where
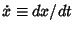 and
and 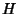 is the so-called Hamiltonian,
is the so-called Hamiltonian,  are called Hamilton's equations. These
equations frequently arise in problems of celestial mechanics.
are called Hamilton's equations. These
equations frequently arise in problems of celestial mechanics.  Another
formulation related to Hamilton's equation is
Another
formulation related to Hamilton's equation is
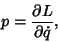 |
(3) |
where 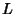 is the so-called Lagrangian.
is the so-called Lagrangian. 
References
Morse, P. M. and Feshbach, H. ``Hamilton's Principle and Classical Dynamics.'' §3.2 in
Methods of Theoretical Physics, Part I. New York: McGraw-Hill, pp. 280-301, 1953.
© 1996-9 Eric W. Weisstein
1999-05-25