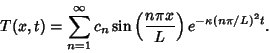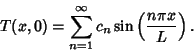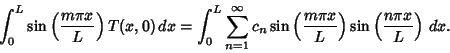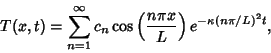A diffusion equation  of the form
of the form
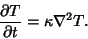 |
(1) |
Physically, the equation commonly arises in situations where 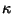 is the
thermal diffusivity
is the
thermal diffusivity  and
and 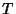 the temperature.
the temperature. 
The 1-D heat conduction equation is
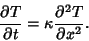 |
(2) |
This can be solved by Separation of Variables using
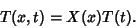 |
(3) |
Then
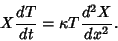 |
(4) |
Dividing both sides by 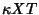 gives
gives
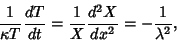 |
(5) |
where each side must be equal to a constant. Anticipating the exponential solution in 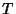 , we have picked a negative
separation constant so that the solution remains finite at all times and
, we have picked a negative
separation constant so that the solution remains finite at all times and 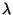 has units of length. The
has units of length. The 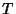 solution
is
solution
is
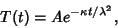 |
(6) |
and the 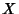 solution is
solution is
 |
(7) |
The general solution is then
If we are given the boundary conditions
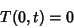 |
(9) |
and
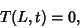 |
(10) |
then applying (9) to (8) gives
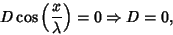 |
(11) |
and applying (10) to (8) gives
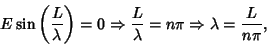 |
(12) |
so (8) becomes
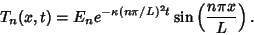 |
(13) |
Since the general solution can have any 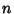 ,
,
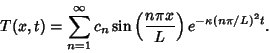 |
(14) |
Now, if we are given an initial condition 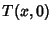 , we have
, we have
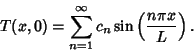 |
(15) |
Multiplying both sides by
 and integrating from 0 to
and integrating from 0 to 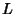 gives
gives
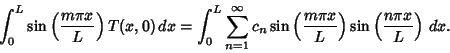 |
(16) |
Using the Orthogonality of 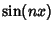 and
and 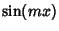 ,
,
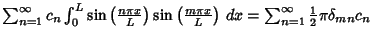
|
|
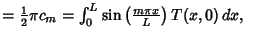
|
(17) |
so
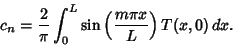 |
(18) |
If the boundary conditions are replaced by the requirement that the derivative of the temperature be zero at the edges,
then (9) and (10) are replaced by
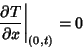 |
(19) |
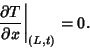 |
(20) |
Following the same procedure as before, a similar answer is found, but with sine replaced by cosine:
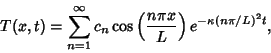 |
(21) |
where
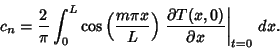 |
(22) |
© 1996-9 Eric W. Weisstein
1999-05-25
![]() of the form
of the form