|
|
|
A Determinant which arises in the solution of the second-order Ordinary Differential Equation
| (1) |
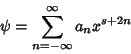 |
(2) |
| (3) |
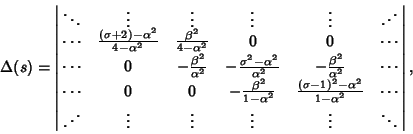 |
(4) |
| (5) | |||
| (6) | |||
| (7) |
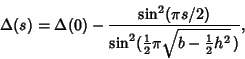 |
(8) |
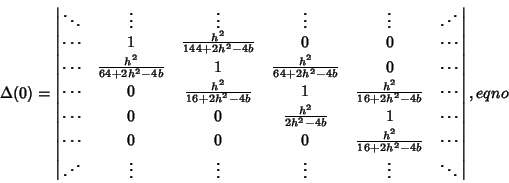 |
(9) |
leading to the implicit equation for ![]() ,
,
| (10) |
See also Hill's Differential Equation
References
Morse, P. M. and Feshbach, H. Methods of Theoretical Physics, Part I. New York:
McGraw-Hill, pp. 555-562, 1953.