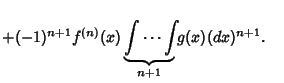A first-order (single) integration by parts uses
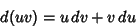 |
(1) |
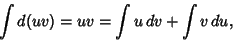 |
(2) |
so
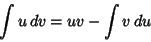 |
(3) |
and
![\begin{displaymath}
\int_a^b u\,dv = [uv]_a^b -\int_{f(a)}^{f(b)}v\,du.
\end{displaymath}](i_817.gif) |
(4) |
Now apply this procedure 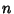 times to
times to
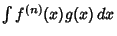 .
.
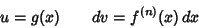 |
(5) |
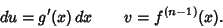 |
(6) |
Therefore,
 |
(7) |
But
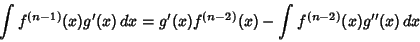 |
(8) |
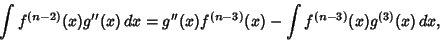 |
(9) |
so
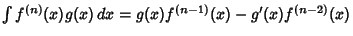
|
|
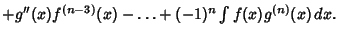
|
(10) |
Now consider this in the slightly different form
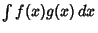 . Integrate by parts a first time
. Integrate by parts a first time
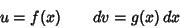 |
(11) |
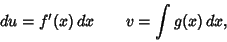 |
(12) |
so
![\begin{displaymath}
\int f(x)g(x)\,dx = f(x)\int g(x)\,dx -\int \left[{\int g(x)\,dx}\right]f'(x)\,dx.
\end{displaymath}](i_829.gif) |
(13) |
Now integrate by parts a second time,
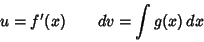 |
(14) |
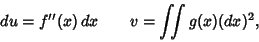 |
(15) |
so
Repeating a third time,
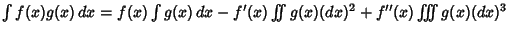
|
|
![$ - \int \left[{\int\!\!\!\int\!\!\!\int g(x)(dx)^3}\right]f'''(x)\,dx.\quad$](i_837.gif)
|
(17) |
Therefore, after 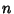 applications,
applications,
If
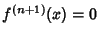 (e.g., for an
(e.g., for an 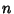 th degree Polynomial), the last term is 0, so the sum terminates after
th degree Polynomial), the last term is 0, so the sum terminates after 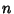 terms and
terms and
References
Abramowitz, M. and Stegun, C. A. (Eds.).
Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing.
New York: Dover, p. 12, 1972.
© 1996-9 Eric W. Weisstein
1999-05-26
![\begin{displaymath}
\int_a^b u\,dv = [uv]_a^b -\int_{f(a)}^{f(b)}v\,du.
\end{displaymath}](i_817.gif)
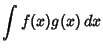
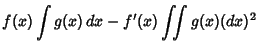
![$\displaystyle + \int \left[{\int\!\!\!\int g(x)(dx)^2}\right]f''(x)\,dx.$](i_835.gif)
![]() applications,
applications,
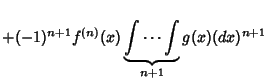
![$+(-1)^{n}\int\left[{\underbrace{\int \cdots \int\!\!}_{n+1} g(x)(dx)^{n+1}}\right]f^{(n+1)}(x)\,dx.\quad$](i_840.gif)