|
|
|
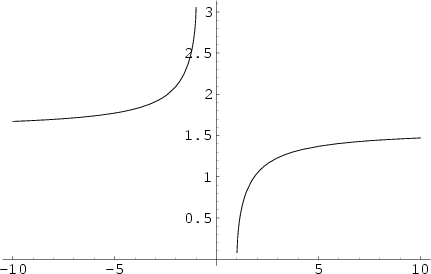

The function ![]() , where
, where ![]() is the Secant and the superscript
is the Secant and the superscript ![]() denotes the Inverse Function,
not the multiplicative inverse. The inverse secant satisfies
denotes the Inverse Function,
not the multiplicative inverse. The inverse secant satisfies
| (1) |
| (2) |
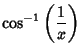 |
(3) | ||
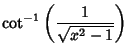 |
(4) | ||
| (5) | |||
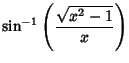 |
(6) | ||
| (7) |
See also Inverse Cosecant, Secant
References
Beyer, W. H. CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, pp. 141-143, 1987.