The jinc function is defined as
where 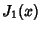 is a Bessel Function of the First Kind, and satisfies
is a Bessel Function of the First Kind, and satisfies
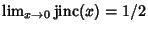 . The
Derivative of the jinc function is given by
. The
Derivative of the jinc function is given by
The function is sometimes normalized by multiplying by a factor of 2 so that
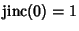 (Siegman 1986, p. 729).
(Siegman 1986, p. 729).
See also Bessel Function of the First Kind, Sinc Function
References
Siegman, A. E. Lasers. Sausalito, CA: University Science Books, 1986.
© 1996-9 Eric W. Weisstein
1999-05-25
