where
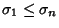 are Lyapunov Characteristic Exponents and
are Lyapunov Characteristic Exponents and 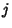 is the largest Integer for
which
is the largest Integer for
which
If 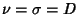 , where
, where 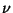 is the Correlation Exponent,
is the Correlation Exponent, 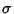 the Information Dimension, and
the Information Dimension, and
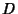 the Hausdorff Dimension, then
the Hausdorff Dimension, then
(Grassberger and Procaccia 1983).
References
Grassberger, P. and Procaccia, I. ``Measuring the Strangeness of Strange Attractors.''
Physica D 9, 189-208, 1983.
© 1996-9 Eric W. Weisstein
1999-05-26