Kappa Curve

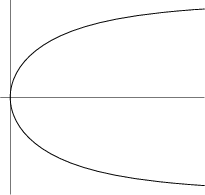
$\begin{figure}\begin{center}\BoxedEPSF{kappa_curve.epsf scaled 700}\end{center}\end{figure}$

A curve also known as Gutschoven's Curve which was first studied by G. van Gutschoven around 1662 (MacTutor Archive). It was also studied by Newton and, some years later, by Johann Bernoulli. It is given by the Cartesian equation

$\begin{displaymath} (x^2+y^2)y^2=a^2 x^2, \end{displaymath}$

(1)

by the polar equation

$\begin{displaymath} r=a\cot\theta, \end{displaymath}$

(2)

and the parametric equations

$\displaystyle x$	$\textstyle =$	$\displaystyle a\cos t\cot t$	(3)
$\displaystyle y$	$\textstyle =$	$\displaystyle a\cos t.$	(4)

References

Lawrence, J. D. A Catalog of Special Plane Curves. New York: Dover, pp. 136 and 139-141, 1972.

MacTutor History of Mathematics Archive. ``Kappa Curve.'' http://www-groups.dcs.st-and.ac.uk/~history/Curves/Kappa.html.