|
|
|
For an infinite population with Mean ![]() , Variance
, Variance ![]() , Skewness
, Skewness ![]() , and
Kurtosis
, and
Kurtosis ![]() , the corresponding quantities for the distribution of means are
, the corresponding quantities for the distribution of means are
| (1) | |||
| (2) | |||
| (3) | |||
| (4) |
| (5) | |||
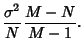 |
(6) |
References
Kenney, J. F. and Keeping, E. S. Mathematics of Statistics, Pt. 1, 3rd ed. Princeton, NJ: Van Nostrand, 1962.