A variable 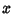 is memoryless with respect to
is memoryless with respect to 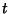 if, for all
if, for all  with
with  ,
,
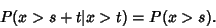 |
(1) |
Equivalently,
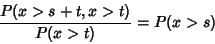 |
(2) |
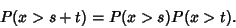 |
(3) |
The Exponential Distribution, which satisfies
and therefore
is the only memoryless random distribution.
See also Exponential Distribution
© 1996-9 Eric W. Weisstein
1999-05-26
![]() is memoryless with respect to
is memoryless with respect to ![]() if, for all
if, for all ![]() with
with ![]() ,
,