The Elliptic Curve
given in Weierstraß Form as
The complete set of solutions to this equation consists of
 , (
, (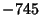 , 4520), (
, 4520), (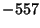 , 13356),
(
, 13356),
(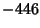 , 14616), (
, 14616), (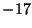 , 10656), (91, 8172), (227, 4228), (247, 3528), (271, 2592), (455, 200), (499, 3276), (523, 4356),
(530, 4660), (599, 7576), (751, 14112), (1003, 25956), (1862, 75778), (3511, 204552), (5287, 381528), (23527, 3607272),
(64507, 16382772), (100102, 31670478), and (1657891, 2134685628) (Stroeker and de Weger 1994).
, 10656), (91, 8172), (227, 4228), (247, 3528), (271, 2592), (455, 200), (499, 3276), (523, 4356),
(530, 4660), (599, 7576), (751, 14112), (1003, 25956), (1862, 75778), (3511, 204552), (5287, 381528), (23527, 3607272),
(64507, 16382772), (100102, 31670478), and (1657891, 2134685628) (Stroeker and de Weger 1994).
References
Guy, R. K. ``The Ochoa Curve.'' Crux Math. 16, 65-69, 1990.
Ochoa Melida, J. ``La ecuacion diofántica
 .'' Gaceta Math. 139-141, 1978.
.'' Gaceta Math. 139-141, 1978.
Stroeker, R. J. and de Weger, B. M. M. ``On Elliptic Diophantine Equations that Defy Thue's Method:
The Case of the Ochoa Curve.'' Experiment. Math. 3, 209-220, 1994.
© 1996-9 Eric W. Weisstein
1999-05-26
![]() .'' Gaceta Math. 139-141, 1978.
.'' Gaceta Math. 139-141, 1978.