|
|
|
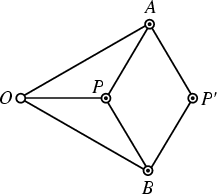
A Linkage with six rods which draws the inverse of a given curve. When a pencil is placed at ![]() , the inverse is drawn
at
, the inverse is drawn
at ![]() (or vice versa). If a seventh rod (dashed) is added (with an additional pivot),
(or vice versa). If a seventh rod (dashed) is added (with an additional pivot), ![]() is kept on a circle and the locus
traced out by
is kept on a circle and the locus
traced out by ![]() is a straight line. It therefore converts circular motion to linear motion without sliding, and was
discovered in 1864. Another Linkage which performs this feat using hinged squares had been published by Sarrus in 1853
but ignored. Coxeter (1969, p. 428) shows that
is a straight line. It therefore converts circular motion to linear motion without sliding, and was
discovered in 1864. Another Linkage which performs this feat using hinged squares had been published by Sarrus in 1853
but ignored. Coxeter (1969, p. 428) shows that
See also Hart's Inversor, Linkage
References
Bogomolny, A. ``Peaucellier Linkage.''
http://www.cut-the-knot.com/pythagoras/invert.html.
Courant, R. and Robbins, H. What is Mathematics?: An Elementary Approach to Ideas and Methods. Oxford, England: Oxford
University Press, p. 156, 1978.
Coxeter, H. S. M. Introduction to Geometry, 2nd ed. New York: Wiley, pp. 82-83, 1969.
Ogilvy, C. S. Excursions in Geometry. New York: Dover, pp. 46-48, 1990.
Rademacher, H. and Toeplitz, O. The Enjoyment of Mathematics: Selections from Mathematics for the Amateur.
Princeton, NJ: Princeton University Press, pp. 121-126, 1957.
Smith, D. E. A Source Book in Mathematics. New York: Dover, p. 324, 1994.