Perfect Ruler

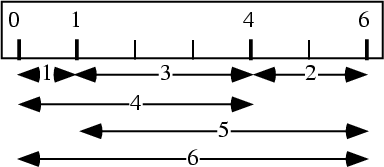
A type of Ruler considered by Guy (1994) which has $k$ distinct marks spaced such that the distances between marks can be used to measure all the distances 1, 2, 3, 4, ... up to some maximum distance $n>k$. Such a ruler can be constructed from a Perfect Difference Set by subtracting one from each element. For example, the Perfect Difference Set $\{1, 2, 5, 7\}$ gives 0, 1, 4, 6, which can be used to measure $1-0=1$, $6-4=2$, $4-1=3$, $4-0=4$, $6-1=5$, $6-0=6$ (so we get 6 distances with only four marks).

See also Perfect Difference Set

References

Guy, R. K. ``Modular Difference Sets and Error Correcting Codes.'' §C10 in Unsolved Problems in Number Theory, 2nd ed. New York: Springer-Verlag, pp. 118-121, 1994.