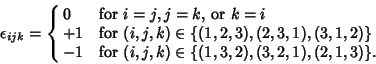A three-index object sometimes called the Levi-Civita Symbol defined by
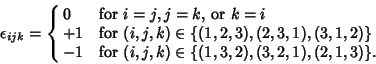 |
(1) |
The permutation symbol satisfies
where  is the Kronecker Delta. The symbol can be defined as the Scalar Triple Product of unit
vectors in a right-handed coordinate system,
is the Kronecker Delta. The symbol can be defined as the Scalar Triple Product of unit
vectors in a right-handed coordinate system,
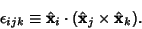 |
(6) |
The symbol can also be interpreted as a Tensor, in which case it is called the Permutation Tensor.
See also Permutation Tensor
References
Arfken, G. Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 132-133, 1985.
© 1996-9 Eric W. Weisstein
1999-05-26