The q-Analog of Pi 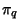 can be defined by taking
can be defined by taking 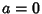 in the
q-Factorial
in the
q-Factorial
giving
where 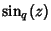 is the q-Sine. Gosper has developed an iterative algorithm for computing
is the q-Sine. Gosper has developed an iterative algorithm for computing 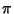 based on the algebraic Recurrence Relation
based on the algebraic Recurrence Relation
© 1996-9 Eric W. Weisstein
1999-05-25
![]() can be defined by taking
can be defined by taking ![]() in the
q-Factorial
in the
q-Factorial