|
|
|
An Auxiliary Latitude which gives a sphere having correct distances along the meridians. It is denoted ![]() (or
(or
![]() ) and is given by
) and is given by
| (1) |
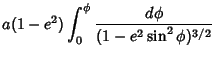 |
|||
![$\displaystyle a\left[{\int_0^\phi \sqrt{1-e^2\sin^2\phi}\,d\phi-{e^2\sin\phi\cos\phi\over\sqrt{1-e^2\sin^2\phi}}}\right].$](r_916.gif) |
|||
| (2) |
| (3) |
| (4) |
| (5) |
| (6) |
See also Latitude
References
Adams, O. S. ``Latitude Developments Connected with Geodesy and Cartography with Tables, Including a Table for
Lambert Equal-Area Meridional Projections.'' Spec. Pub. No. 67. U. S. Coast and Geodetic Survey, pp. 125-128, 1921.
Snyder, J. P. Map Projections--A Working Manual. U. S. Geological Survey Professional Paper 1395. Washington,
DC: U. S. Government Printing Office, pp. 16-17, 1987.
|
|
|
© 1996-9 Eric W. Weisstein