|
|
|
A special class of Enneper's Negative Curvature Surfaces which can be given parametrically by
| (1) | |||
| (2) | |||
| (3) |
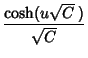 |
(4) | ||
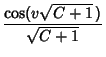 |
(5) | ||
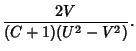 |
(6) |
| (7) |
See also Enneper's Negative Curvature Surfaces, Kuen Surface, Sievert's Surface
References
Fischer, G. (Ed.). Plate 88 in
Mathematische Modelle/Mathematical Models, Bildband/Photograph Volume. Braunschweig, Germany: Vieweg, p. 84, 1986.
Reckziegel, H. ``Sievert's Surface.'' §3.4.4.3 in
Mathematical Models from the Collections of Universities and Museums (Ed. G. Fischer).
Braunschweig, Germany: Vieweg, pp. 39-40, 1986.
Rembs, E. ``Enneper'sche Flächen konstanter positiver Krümmung und Hazzidakissche Transformationen.''
Jahrber. DMV 39, 278-283, 1930.