|
|
|
N.B. A detailed on-line essay by S. Finch was the starting point for this entry.
Let the number of Random Walks on a ![]() -D lattice starting at the Origin which never land on
the same lattice point twice in
-D lattice starting at the Origin which never land on
the same lattice point twice in ![]() steps be denoted
steps be denoted ![]() . The first few values are
. The first few values are
| (1) | |||
| (2) | |||
| (3) |
| (4) |
| (5) | |||
| (6) | |||
| (7) | |||
| (8) | |||
| (9) |
For the triangular lattice in the plane, ![]() (Alm 1993), and for the hexagonal planar lattice, it is conjectured that
(Alm 1993), and for the hexagonal planar lattice, it is conjectured that
| (10) |
The following limits are also believed to exist and to be Finite:
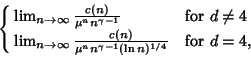 |
(11) |
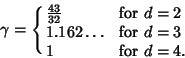 |
(12) |
Define the mean square displacement over all ![]() -step self-avoiding walks
-step self-avoiding walks ![]() as
as
| (13) |
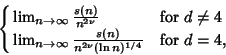 |
(14) |
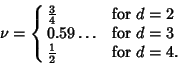 |
(15) |
See also Random Walk
References
Alm, S. E. ``Upper Bounds for the Connective Constant of Self-Avoiding Walks.'' Combin. Prob. Comput. 2, 115-136, 1993.
Finch, S. ``Favorite Mathematical Constants.'' http://www.mathsoft.com/asolve/constant/cnntv/cnntv.html
Madras, N. and Slade, G. The Self-Avoiding Walk. Boston, MA: Birkhäuser, 1993.
|
|
|
© 1996-9 Eric W. Weisstein