|
|
|
The Somos sequences are a set of related symmetrical Recurrence Relations which,
surprisingly, always give integers. The Somos sequence of order ![]() is defined by
is defined by
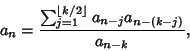
|
|
|
|
|
|
|
|
|
|
|
However, the ![]() -Somos sequences for
-Somos sequences for ![]() do not give integers. The values of
do not give integers. The values of ![]() for which
for which ![]() first
becomes nonintegral for the
first
becomes nonintegral for the ![]() -Somos sequence for
-Somos sequence for ![]() , 9, ... are
17, 19, 20, 22, 24, 27, 28, 30, 33, 34, 36, 39, 41, 42, 44, 46, 48, 51, 52, 55, 56, 58, 60, ...
(Sloane's A030127).
, 9, ... are
17, 19, 20, 22, 24, 27, 28, 30, 33, 34, 36, 39, 41, 42, 44, 46, 48, 51, 52, 55, 56, 58, 60, ...
(Sloane's A030127).
See also Göbel's Sequence, Heronian Triangle
References
Buchholz, R. H. and Rathbun, R. L. ``An Infinite Set of Heron Triangles with Two Rational Medians.''
Amer. Math. Monthly 104, 107-115, 1997.
Gale, D. ``Mathematical Entertainments: The Strange and Surprising Saga of the
Somos Sequences.'' Math. Intel. 13, 40-42, 1991.
Sloane, N. J. A. Sequences
A030127,
A006720/M0857,
A006721/M0735,
A006722/M2457,
A006723/M2456
in ``An On-Line Version of the Encyclopedia of Integer Sequences.''
http://www.research.att.com/~njas/sequences/eisonline.html and Sloane, N. J. A. and Plouffe, S.
The Encyclopedia of Integer Sequences. San Diego: Academic Press, 1995.
|
|
|
© 1996-9 Eric W. Weisstein