|
|
|
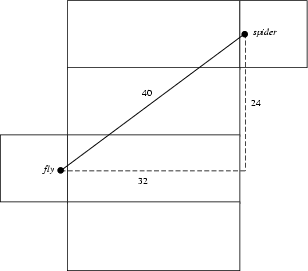
In a rectangular room (a Cuboid) with dimensions
![]() , a spider is located in the middle of one
, a spider is located in the middle of one
![]() wall one foot away from the ceiling. A fly is in the middle of the opposite wall one foot away from the
floor. If the fly remains stationary, what is the shortest distance the spider must crawl to capture the fly? The answer,
wall one foot away from the ceiling. A fly is in the middle of the opposite wall one foot away from the
floor. If the fly remains stationary, what is the shortest distance the spider must crawl to capture the fly? The answer,
![]() , can be obtained by ``flattening'' the walls as illustrated above.
, can be obtained by ``flattening'' the walls as illustrated above.
References
Pappas, T. ``The Spider & the Fly Problem.'' The Joy of Mathematics.
San Carlos, CA: Wide World Publ./Tetra, pp. 218 and 233, 1989.