|
|
|
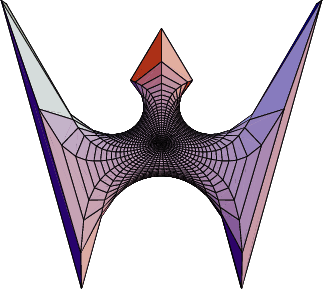
A Minimal Surface discovered by L. P. M. Jorge and W. Meeks III in 1983 with Enneper-Weierstraß
Parameterization
| (1) | |||
| (2) |
![$\displaystyle \Re\left[{{r e^{i\theta}\over 3(1 + r e^{i\theta} + r^2 e^{2i\the...
...\theta}-1)\over 9} + {2\ln(1 + r e^{i\theta} + r^2e^{2i\theta})\over 9}}\right]$](t_2224.gif) |
(3) | ||
![$\displaystyle -{\textstyle{1\over 9}}\Im\left[{{-3re^{i\theta}(1+re^{i\theta})\...
...-1} \left({1+2re^{i\theta}\over\sqrt{3}}\right)\over r^3e^{3i\theta}-1}}\right]$](t_2225.gif) |
(4) | ||
![$\displaystyle \Re\left[{-{2\over 3} - {2\over 3(r^3 e^{3i\theta}-1)}}\right],$](t_2226.gif) |
(5) |
See also Minimal Surface
References
Dickson, S. ``Minimal Surfaces.'' Mathematica J. 1, 38-40, 1990.
![]() Wolfram Research ``Mathematica Version 2.0 Graphics Gallery.''
http://www.mathsource.com/cgi-bin/MathSource/Applications/Graphics/3D/0207-155.
Wolfram Research ``Mathematica Version 2.0 Graphics Gallery.''
http://www.mathsource.com/cgi-bin/MathSource/Applications/Graphics/3D/0207-155.