|
|
|
A Set of Positive integers is called weakly triple-free if, for any integer ![]() , the Set
, the Set
![]() . It is called strongly triple-free if
. It is called strongly triple-free if ![]() Implies
Implies ![]() and
and ![]() . Define
. Define
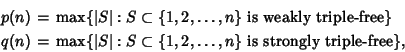
See also Double-Free Set
References
Finch, S. ``Favorite Mathematical Constants.'' http://www.mathsoft.com/asolve/constant/triple/triple.html