Let 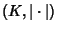 be a non-Archimedean valuated field. Its valuation ring
be a non-Archimedean valuated field. Its valuation ring 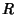 is defined to be
is defined to be
The valuation ring has maximal Ideal
and the field 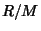 is called the residue field, class field, or field of digits. For example, if
is called the residue field, class field, or field of digits. For example, if 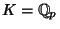 (
(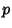 -adic numbers), then
-adic numbers), then 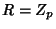 (
(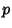 -adic integers),
-adic integers), 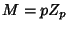 (
(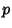 -adic integers congruent to 0 mod
-adic integers congruent to 0 mod 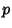 ), and
), and
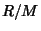 = GF(
= GF(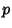 ), the Finite Field of order
), the Finite Field of order 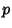 .
.
© 1996-9 Eric W. Weisstein
1999-05-26
![]() be a non-Archimedean valuated field. Its valuation ring
be a non-Archimedean valuated field. Its valuation ring ![]() is defined to be
is defined to be