An identity in Calculus of Variations discovered in 1868 by Beltrami. The Euler-Lagrange Differential
Equation is
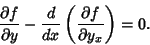 |
(1) |
Now, examine the Derivative of 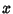
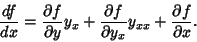 |
(2) |
Solving for the
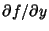 term gives
term gives
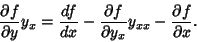 |
(3) |
Now, multiplying (1) by 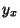 gives
gives
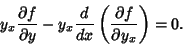 |
(4) |
Substituting (3) into (4) then gives
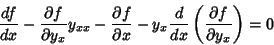 |
(5) |
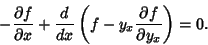 |
(6) |
This form is especially useful if 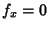 , since in that case
, since in that case
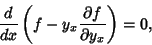 |
(7) |
which immediately gives
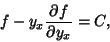 |
(8) |
where 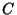 is a constant of integration.
is a constant of integration.
The Beltrami identity greatly simplifies the solution for the minimal Area Surface of Revolution about a given
axis between two specified points. It also allows straightforward solution of the Brachistochrone Problem.
See also Brachistochrone Problem, Calculus of Variations, Euler-Lagrange Differential Equation,
Surface of Revolution
© 1996-9 Eric W. Weisstein
1999-05-26