The 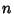 th Beraha constant is given by
th Beraha constant is given by
The first few are
They appear to be Roots of the Chromatic Polynomials of planar triangular
Graphs. 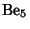 is
is 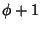 , where
, where 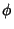 is the Golden Ratio, and
is the Golden Ratio, and 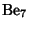 is the Silver Constant.
is the Silver Constant.
References
Le Lionnais, F. Les nombres remarquables. Paris: Hermann, p. 143, 1983.
© 1996-9 Eric W. Weisstein
1999-05-26
![]() th Beraha constant is given by
th Beraha constant is given by