|
|
|
The boustrophedon (``ox-plowing'') transform b of a sequence a is given by
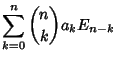 |
(1) | ||
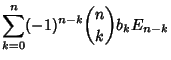 |
(2) |
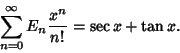 |
(3) |
| (4) |
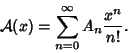 |
(5) |
See also Alternating Permutation, Entringer Number, Secant Number, Seidel-Entringer-Arnold Triangle, Tangent Number
References
Millar, J.; Sloane, N. J. A.; and Young, N. E. ``A New Operation on Sequences: The Boustrophedon Transform.''
J. Combin. Th. Ser. A 76, 44-54, 1996.