|
|
|
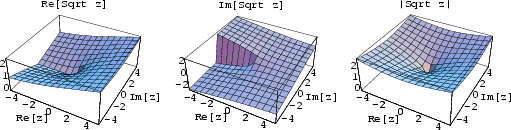
A line in the Complex Plane across which a Function is discontinuous.
| function | branch cut(s) |
|
|
|
|
|
|
|
|
See also Branch Point
References
Morse, P. M. and Feshbach, H. Methods of Theoretical Physics, Part I. New York:
McGraw-Hill, pp. 399-401, 1953.