|
|
|
Let the probabilities of various classes in a distribution be ![]() ,
, ![]() ,
...,
,
..., ![]() . The expected frequency
. The expected frequency
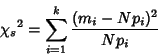
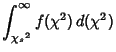 |
|||
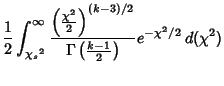 |
|||
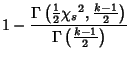 |
|||
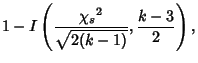 |
When fitting a one-parameter solution using ![]() , the best-fit parameter value can be found by calculating
, the best-fit parameter value can be found by calculating ![]() at
three points, plotting against the parameter values of these points, then finding the minimum of a Parabola fit through
the points (Cuzzi 1972, pp. 162-168).
at
three points, plotting against the parameter values of these points, then finding the minimum of a Parabola fit through
the points (Cuzzi 1972, pp. 162-168).
References
Cuzzi, J. The Subsurface Nature of Mercury and Mars from Thermal Microwave Emission. Ph.D. Thesis. Pasadena, CA:
California Institute of Technology, 1972.
Kenney, J. F. and Keeping, E. S. Mathematics of Statistics, Pt. 2, 2nd ed. Princeton, NJ: Van Nostrand, 1951.