|
|
|
Functions which can be expressed in terms of Legendre Functions of the First and Second Kinds. See Abramowitz and Stegun (1972, p. 337).
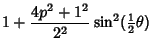 |
|||
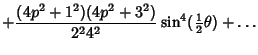 |
|||
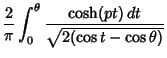 |
|||
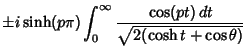 |
|||
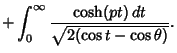 |
See also Toroidal Function
References
Abramowitz, M. and Stegun, C. A. (Eds.). ``Conical Functions.''
§8.12 in Handbook of Mathematical Functions with Formulas,
Graphs, and Mathematical Tables, 9th printing. New York: Dover, p. 337, 1972.
Iyanaga, S. and Kawada, Y. (Eds.). Encyclopedic Dictionary of Mathematics.
Cambridge, MA: MIT Press, p. 1464, 1980.