|
|
|
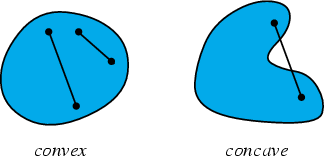
A Set in Euclidean Space ![]() is convex if it contains all the Line Segments
connecting any pair of its points. If the Set does not contain all the Line Segments, it is
called Concave.
is convex if it contains all the Line Segments
connecting any pair of its points. If the Set does not contain all the Line Segments, it is
called Concave.
See also Connected Set, Convex Function, Convex Hull, Convex Optimization Theory, Convex Polygon, Delaunay Triangulation, Minkowski Convex Body Theorem, Simply Connected
References
Croft, H. T.; Falconer, K. J.; and Guy, R. K. ``Convexity.'' Ch. A in
Unsolved Problems in Geometry. New York: Springer-Verlag, pp. 6-47, 1994.